1 min read Papers

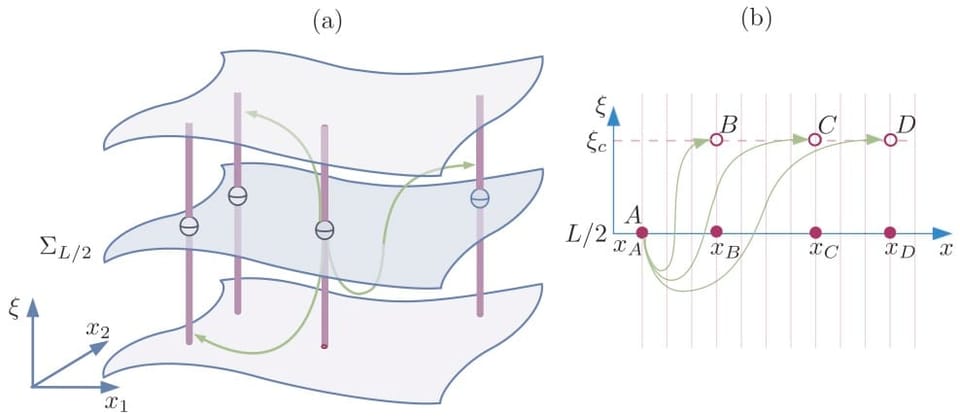
Paper on well-posedness of neural fields with anisotropic diffusion

Paper on well-posedness of neural fields with anisotropic diffusion
A novel modelling strategy for cortical activity describes the cortex as a heterogeneous medium: a middle layer contains cellular nuclei, while neurons stretch along vertical dendritic fibres. Neurons connects the somatic layer to a contact point on the fibres.

A new paper by Amsterdam Dynamics member Daniele Avitabile, and collaborators Nikolai Chemetov and Pedro Lima studies well-posedness of solutions to a novel type of neural field model. Standard neural field models do not distinguish between somas (containing the nucleus of a neuron) and dendrites. The new model features dendrites as vertically aligned fibres, along which the voltage propagates by diffusion. The paper studies the existence, uniqueness, and regularity of solutions to the neural field with anisotropic diffusion, and derives rigorous asymptotic estimates valid in the small diffusion limit. These results are relevant to compute numerical solutions to this neural field type.