New preprint proves existence of quasi-patterns in spatially extended PDEs

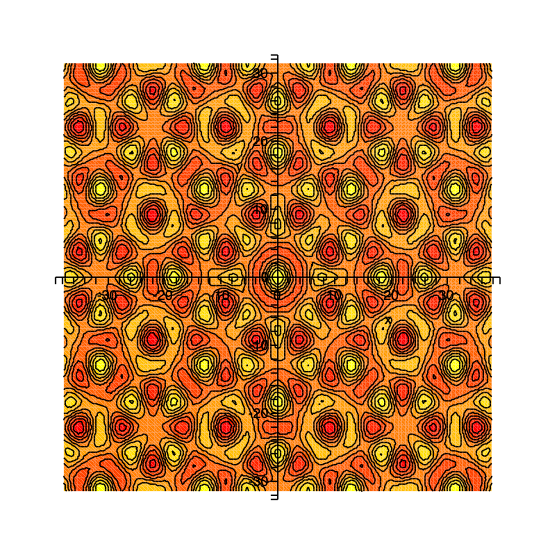
Quasi-patterns are symmetric solutions of spatially extended PDEs which are non-periodic but have a discrete diffraction diagram. Such patterns have been found in fluid experiments such as the Faraday wave experiment and in shaken convection, and have received a lot of interest since the 1980. Well-known examples include planar quasi-patterns with higher order rotational symmetry, and spatial quasi-patterns with icosahedral symmetry.

A recent preprint by Ian Melbourne (Warwick), Jens Rademacher (Hamburg), Bob Rink (VU) and Sergey Zelik (Surrey) shows that the existence of quasi-patterns is a natural consequence of spontaneous symmetry breaking. Exploiting recent regularity results for almost-periodic solutions of parabolic PDEs, the authors also prove the all-time existence of quasi-pattern solutions to the well-known Swift-Hohenberg equation.